ecuaciones bicuadradas

|

Resuelve la ecuación: x^4-8x^2-9=0

SOLUCIÓN

x^4-8x^2-9=0


Se trata de una ecuación bicuadrada: ecuación de 4º grado que no tiene término en x ni término en x^3.
Para resolverla por el método de las bicuadradas tenemos que hacer el siguiente cambio de variable:
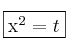\fbox{x^2=t} con lo cual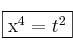 \fbox{x^4=t^2}

x^4-8x^2-9=0


t^2-8t-9=0


Resolvemos la ecuación de 2º grado en la variable "t"

\begin{array}{ccc} & & t_1 = \frac{8+10}{2}=9\\ & \nearrow &\\ t=\frac{-(-8)\pm \sqrt{(-8)^2-4 \cdot1\cdot(-9)}}{2 \cdot1}= \frac{8\pm \sqrt{100}}{2}& &\\ & \searrow &\\& &t_2 = \frac{8-10}{2}=-1\end{array}


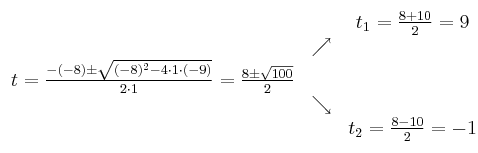
Ahora deshacemos el cambio de variable

x^2=t \longrightarrow x = \pm \sqrt{t}


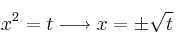
– Si t=9 \longrightarrow x= \pm \sqrt{9} \longrightarrow x= \pm 3
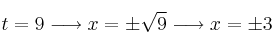
– Si t=-1 \longrightarrow x= \pm \sqrt{-1} sin soluciones reales

Las soluciones de la ecuación son 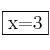\fbox{x=3} y \fbox{x=-3}