funciones
SOLUCIÓN
Para hallar los puntos de corte con los ejes de coordenadas seguimos estos pasos:
– 1) Hacemos ![]() y calculamos
y calculamos ![]() . Nos dará los puntos de corte con el eje de ordenadas (eje vertical)
. Nos dará los puntos de corte con el eje de ordenadas (eje vertical)
– 2) Hacemos ![]() y calculamos
y calculamos ![]() . Nos dará los puntos de corte con el eje de abcisas (eje horizontal)
. Nos dará los puntos de corte con el eje de abcisas (eje horizontal)
– a) ![]()
Si ![]()
Punto de corte ![]()
Si ![]()
Resolvemos la ecuación de segundo grado

Las soluciones son x=3 y x=-1
Puntos de corte
– b) ![]()
Si ![]()
Punto de corte ![]()
Si ![]()
Resolvemos la ecuación de segundo grado
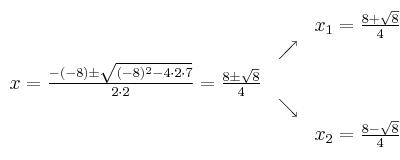
Puntos de corte
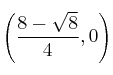 y
y 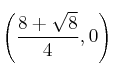
– c) ![]()
Si ![]()
Punto de corte ![]()
Si 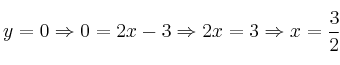
Punto de corte 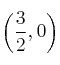
– d) ![]()
Si ![]()
Punto de corte ![]()
Si ![]() Ecuación sin solución
Ecuación sin solución
No hay más puntos de corte
La función constante ![]() es una recta horizontal que pasa por el punto
es una recta horizontal que pasa por el punto ![]() , por tanto no corta al eje de abcisas (eje horizontal)
, por tanto no corta al eje de abcisas (eje horizontal)
 Matemáticas IES
Matemáticas IES