funciones
SOLUCIÓN
– a) Calcula tres puntos de esa recta
![]()
Si ![]()
Punto: 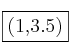
Si ![]()
Punto: 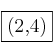
Si ![]()
Punto: 
– b) ¿Pasa por el punto ![]() ? ¿Y por el punto
? ¿Y por el punto ![]() ?
?
Si ![]()
No pasa por el punto ![]()
Si ![]()
Si pasa por el punto ![]()
– c) Indica su pendiente

La pendiente es ![]()
– d) Escribe la ecuación de tres rectas paralelas
Dos rectas son paralelas cuando tienen la misma pendiente.
Rectas paralelas a ![]() serían por ejemplo:
serían por ejemplo:
![]()
![]()
![]()
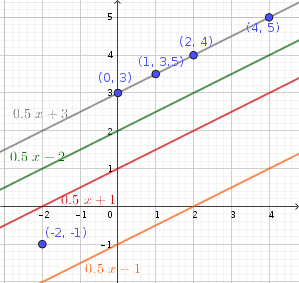
Veamos gráficamente todos los resultados:
 Matemáticas IES
Matemáticas IES