Selectividad Andalucía 2001-3-B3

, por dani

|

– a) \left. \begin{array}{ccc} mx+ y -z & = & 1 \\ x - my+ z & = & 4 \\ x + y+ mz & = & m \end{array} \right\}
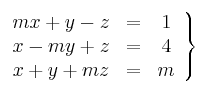
Expresamos la matriz de los coeficiente y la matriz ampliada:

A | A^*= \left( \begin{array}{ccc|c} m & 1 & -1 & 1 \\ 1 & -m & 1 & 4 \\ 1 & 1 & m & m \end{array} \right)

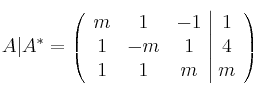
Calculamos determinante de A
|A | = \left| \begin{array}{ccc} m & 1 & -1 \\ 1 & -m & 1 \\ 1 & 1 & m \end{array} \right| = -m^3-3m

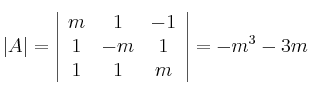
Veamos en qué casos valdrá cero.

|A| = 0 \l \Leftrightarrow -m^3-3m=0 \Leftrightarrow m=0

– Si m \neq 0 \Longrightarrow |A| \neq 0 \Longrightarrow rang(A) = 3
Como rang(A*) = 3 y el número de incógnitas es también 3, el sistema será Compatible Determinado (T. Rouche).

– Si m=0 veamos lo que ocurre:
Sustituimos m por 0 y calculamos los rangos de A y A*

A | A^* = \left( \begin{array}{ccc|c} 0 & 1 & -1 & 1 \\ 1 & 0 & 1 & 4 \\ 1 & 1 & 0 & 0 \end{array} \right)

\left| \begin{array}{cc} 0 & 1 \\ 1 & 0 \end{array} \right| \neq 0 \Longrightarrow rang(A)=2

\left| \begin{array}{ccc} 0 & 1 & 1 \\ 1 & 0 & 4 \\ 1 & 1 & 0 \end{array} \right| \neq 0 \Longrightarrow rang(A*)=3

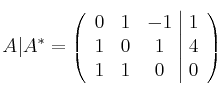
Al ser los rangos distintos, el sistema es Icompatible

Considera el sistema
\left. \begin{array}{ccc} mx+ y -z & = & 1 \\ x - my+ z & = & 4 \\ x + y+ mz & = & m \end{array} \right\}

– a) Discútelo según los valores de m
– b) ¿Cuál es, según los valores de m, la posición relativa de los planos cuyas ecuaciones respectivas son las tres que forman el sistema?