-
Demuestra que  , siendo las matrices
, siendo las matrices
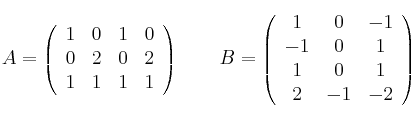
-
Demuestra que  , siendo las matrices
, siendo las matrices
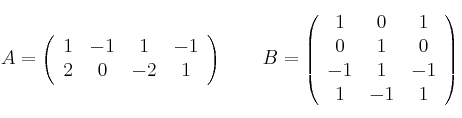
-
Indica si las siguientes matrices son regulares o singulares:

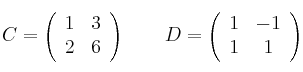
-
Calcula aplicando la Regla de Sarrus el determinante de la siguiente matriz:
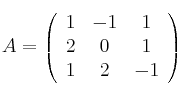
-
Sea la matriz
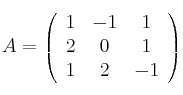
– Calcula su determinante