Estudio Global de Funciones
SOLUCIÓN
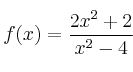
Dominio
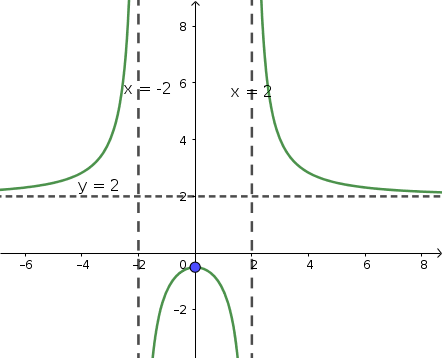
Se trata de una función racional. Su dominio es todos los números excepto los que anulan el denominador.
![]()
![]()
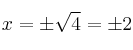
![]()
Corte con los ejes de coordenadas
Si 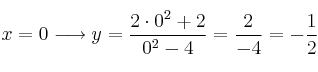
Punto de corte 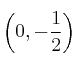
Si 
![]()
No tiene soluciones reales.
No hay puntos de corte en el eje de abcisas (eje horizontal)
Simetrías
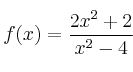
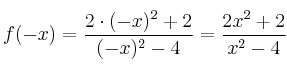
Como ![]() se trata de una función par, es simétrica respecto al eje de ordenadas (eje vertical)
se trata de una función par, es simétrica respecto al eje de ordenadas (eje vertical)
Asíntotas
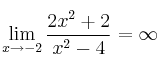
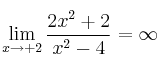
Tiene asíntotas verticales en 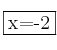 y
y 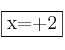
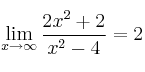
Tiene asíntota horizontal en 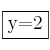
Monotonía
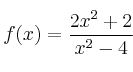
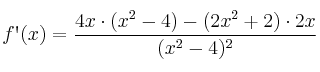

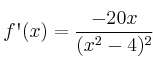
![]()
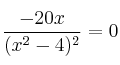
![]()
Las soluciones de la ecuación anterior y los puntos de discontinuidad nos dividen la recta real en varios intervalos
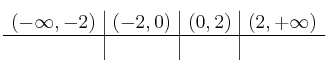
Tomamos un punto de cada intervalo para comprobar el signo de la derivada
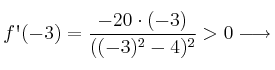 CRECE
CRECE
 CRECE
CRECE
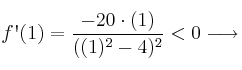 DECRECE
DECRECE
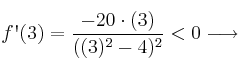 DECRECE
DECRECE
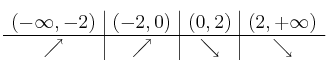
Extremos
Teniendo en cuenta que:
– En x=0 es continua
– Crece a la izquierda de 0
– Decrece a la derecha de 0
Hay un Máximo en x=0
Calculamos la segunda coordenada del máximo
Si 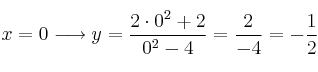
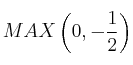
 Matemáticas IES
Matemáticas IES