Estudio Global de Funciones
SOLUCIÓN
Estudio global de ![]()
Dominio
![]()
El dominio de cualquier función polinómica es "todos los números reales"
Asíntotas
Una función polinómica no tiene asíntotas.
Simetrías
![]()
Es una función par (simétrica respecto al eje OY)
Corte con los ejes
– Si ![]() entonces
entonces ![]() . Punto:
. Punto:![]()
– Si ![]() entonces
entonces ![]() . Resolvemos la ecuación:
. Resolvemos la ecuación:
![]() de donde:
de donde:
![]()
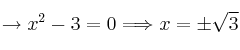
Puntos: ![]() ,
, 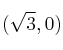 ,
, 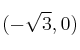
Monotonía
![]()
![]()
De donde:
![]()
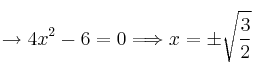
Intervalos:
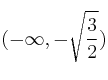 ,
,  ,
, 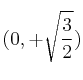 ,
, 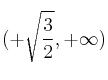
Tomamos un punto de cada intervalo para ver el signo de la derivada (positivo significa creciente y negativo decreciente)
– ![]() DECRECIENTE
DECRECIENTE
– ![]() CRECIENTE
CRECIENTE
– ![]() DECRECIENTE
DECRECIENTE
– ![]() CRECIENTE
CRECIENTE

Extremos
Aplicamos la derivada segunda a los puntos 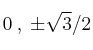
![]()
– ![]() MÁXIMO en
MÁXIMO en ![]()
– 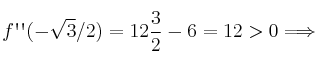 MÍNIMO en
MÍNIMO en 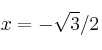
– 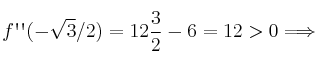 MÍNIMO en
MÍNIMO en 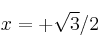
Calculemos la imagen de los extremos para conocer la segunda coordenada:
– ![]()
–  MIN
MIN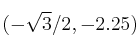
–  MIN
MIN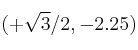
Gráfica
Con los datos obtenidos anteriormente:

podemos ya dibujar la gráfica:

 Matemáticas IES
Matemáticas IES