Ecuaciones grado superior
SOLUCIÓN
Se trata de una generalización del método de las ecuaciones bicuadradas
Hacemos el cambio ![]() y ahora sustituimos
y ahora sustituimos
![]()
Resolvemos la ecuación de segundo grado en la variable "t"
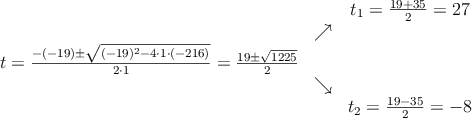
Ahora deshacemos el cambio:
![]()
![]()
![]()
Se trata de una generalización del método de las ecuaciones bicuadradas
Hacemos el cambio ![]() y ahora sustituimos
y ahora sustituimos
![]()
Resolvemos la ecuación de segundo grado en la variable "t"
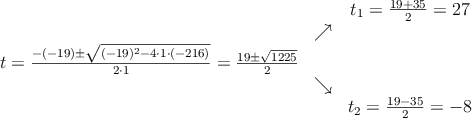
Ahora deshacemos el cambio:
![]()
![]()
![]()